SKEDSOFT

Dirichlet Integral and Fourier Series:

Many of the ideas used in the previous section arise naturally in the basic analysis of Fourier Series. We introduce the basuc definition and then prove a theorem that implicity contains the solution to the Dirchlet integrals.

If f is any function that is integrable on [ -π, π], the numbers

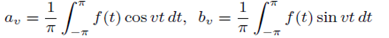
are called the Fourier coeffiecent of f. We can write down the series

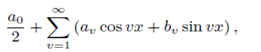
Which is called the Fourier series corresponding to f. We want to know under what condition does the Fourier series corresponding to f actually converge to f. We assume that f is also 2π periodic. Let us consider the partial sums.

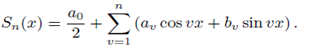
By substituting the expression of the coefficients av and bv found above and interchanging the order of integration and summation we get;

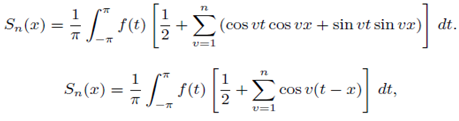
Since,

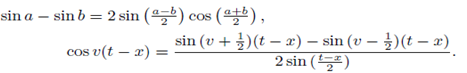
If we sum the last expression from 1 to n, we get;

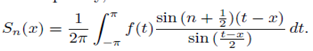
Substitute u = (t-x) and we have;

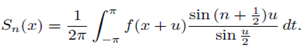
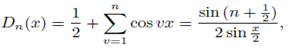
Now we recall that we defined f to be periodically extended beyond the interval [ -π, π]. But for each point at which f (x) ahve a jump discontinuity, we get